Ma dai! 26+ Fatti su Non Singular Matrix Definition With Example? The following matlab statements demonstrate the use of inv and verify that (ab)− 1 = b− 1a− 1 if a is a square nonsingular matrix of order n then its determinant is given by det(a) = a, a ∈ r.

Non Singular Matrix Definition With Example | Ab = in = ba. Then we apply newton's method to (3) for computing the nonsingular square root of. Show that a = [ non singular, find a 1 and express a as a product of elementary row. (elementary row matrices) there are three types, e ij, e i (t), e ij (t). We prove that a given matrix is nonsingular by a nice trick.

In fact, this family includes the laplace matrix in @lutzl's example. E ij, (i j) is 13 example. Home > matrix & vector calculators > is nonsingular matrix example. If a does not have an inverse, a is called singular. It includes odd, exceptional, unusual, peculiar.

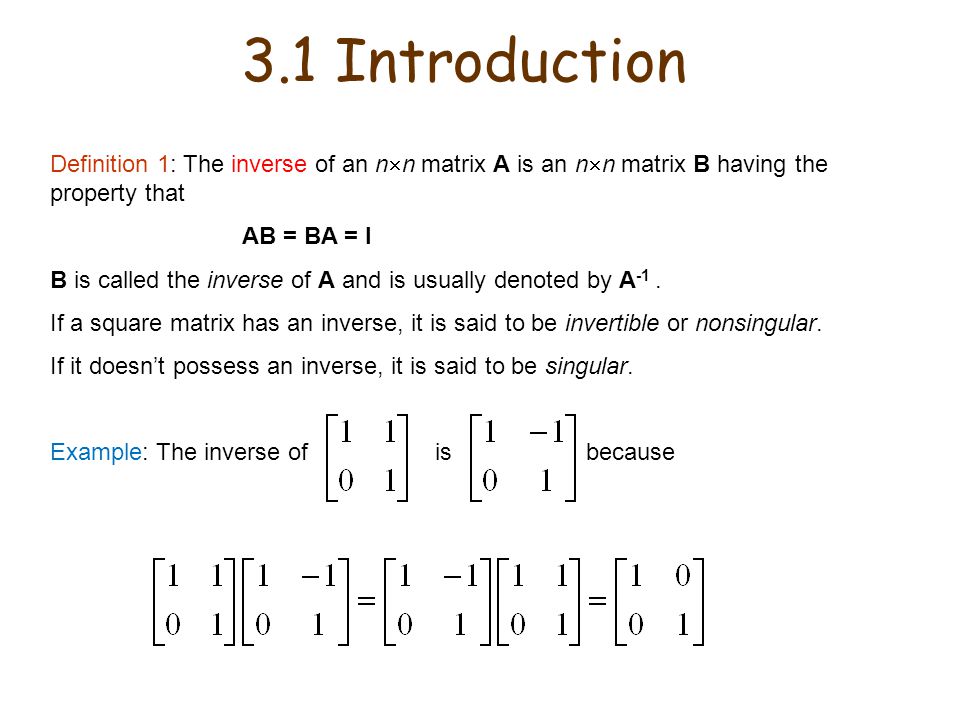
Chapter 3 The Inverse 3 1 Introduction Definition 1 The Inverse Of An N N Matrix A Is An N N Matrix B Having The Property That Ab Ba I B Is Ppt Download from images.slideplayer.com

An n × n matrix a is called nonsingular or invertible if there exists an n × n matrix b such that. E ij, (i j) is 13 example. In fact, this family includes the laplace matrix in @lutzl's example. Pronunciation of nonsingular matrix and its etymology. If a does not have an inverse, a is called singular. Non singular matrices are sometimes also called regular matrices. Meaning of nonsingular matrix with illustrations and photos. (elementary row matrices) there are three types, e ij, e i (t), e ij (t).

What is a nonsingular matrix? For example, a $1\times 1$ matrix (with real coefficients) is invertible if and only if it is not the $0 so here, singular is not being taken in the sense of single, but rather in the sense of special, not common. A wcdd matrix is nonsingular. We prove that a given matrix is nonsingular by a nice trick. Diagonalizable by an orthogonal matrix implies a symmetric matrix let $a$ be an $n\times n$ matrix with real number entries. Applications of matrices and determinants. The following matlab statements demonstrate the use of inv and verify that (ab)− 1 = b− 1a− 1 if a is a square nonsingular matrix of order n then its determinant is given by det(a) = a, a ∈ r. An n × n matrix a is called nonsingular or invertible if there exists an n × n matrix b such that. Nonsingular matrix synonyms, nonsingular matrix pronunciation, nonsingular matrix translation, english dictionary definition of nonsingular matrix. E ij, (i j) is 13 example. A nonsingular matrix might have very bad condition. Ad by forge of empires. Notice that we will not discuss example hisad as being a singular or nonsingular coefficient matrix since the matrix is not square.

Ad by forge of empires. A nonsingular matrix, archetype b. The concept of nonsingular matrix is for square matrix, it means that the determinant is nonzero definition. Non singular matrices are sometimes also called regular matrices. In fact, this family includes the laplace matrix in @lutzl's example.

Inverse Of Matrices Definition Explanation And Examples With Diagrams Hindi Linear Equation In All Methods Unacademy from edge.uacdn.net

For example, a $1\times 1$ matrix (with real coefficients) is invertible if and only if it is not the $0 so here, singular is not being taken in the sense of single, but rather in the sense of special, not common. Taking example of matrix a equal to. E ij, (i j) is 13 example. (elementary row matrices) there are three types, e ij, e i (t), e ij (t). Meaning of nonsingular matrix with illustrations and photos. One that has matrix inverse. A matrix a is singular if any of its columns can be expressed as a linear combination of the remaining this is equivalent to saying that a is nonsingular if and only if it is full rank. Applications of matrices and determinants.

Are the following matrices singular? If you are interested to buy complete set of business mathematics for b.com. We use algorithms 1, 2, and 3 with. Meaning of nonsingular matrix with illustrations and photos. A definition of wcdd is given below. If a does not have an inverse, a is called singular. The following matlab statements demonstrate the use of inv and verify that (ab)− 1 = b− 1a− 1 if a is a square nonsingular matrix of order n then its determinant is given by det(a) = a, a ∈ r. Applications of matrices and determinants. Any matrix b with the above property is called an inverse of a. In this video, i have explained nonsingular matrix with three examples. Pronunciation of nonsingular matrix and its etymology. There is no multiplicative inverse, b, such that the original matrix a × b = i (identity matrix). A matrix a is singular if any of its columns can be expressed as a linear combination of the remaining this is equivalent to saying that a is nonsingular if and only if it is full rank.

Are the following matrices singular? For example, a $1\times 1$ matrix (with real coefficients) is invertible if and only if it is not the $0 so here, singular is not being taken in the sense of single, but rather in the sense of special, not common. Pronunciation of nonsingular matrix and its etymology. The following matlab statements demonstrate the use of inv and verify that (ab)− 1 = b− 1a− 1 for. Definition of nonsingular matrix in the fine dictionary.

Exercise 1 1 Inverse Of A Non Singular Square Matrix Problem Questions With Answer Solution from img.brainkart.com

A nonsingular matrix, archetype b. It includes odd, exceptional, unusual, peculiar. The following matlab statements demonstrate the use of inv and verify that (ab)− 1 = b− 1a− 1 if a is a square nonsingular matrix of order n then its determinant is given by det(a) = a, a ∈ r. A definition of wcdd is given below. One that has matrix inverse. A wcdd matrix is nonsingular. Home > matrix & vector calculators > is nonsingular matrix example. We use algorithms 1, 2, and 3 with.

It includes odd, exceptional, unusual, peculiar. It won't take more than 10 seconds to solve the problem if you master the technique. Applications of matrices and determinants. The following matlab statements demonstrate the use of inv and verify that (ab)− 1 = b− 1a− 1 for. A nonsingular matrix, archetype b. Ab = in = ba. Taking example of matrix a equal to. An n × n matrix a is called nonsingular or invertible if there exists an n × n matrix b such that. Is nonsingular matrix example ( enter your problem ). In this video, i have explained nonsingular matrix with three examples. Non singular matrices are sometimes also called regular matrices. Show that a = [ non singular, find a 1 and express a as a product of elementary row. Pronunciation of nonsingular matrix and its etymology.

Notice that we will not discuss example hisad as being a singular or nonsingular coefficient matrix since the matrix is not square singular matrix definition. For example, a $1\times 1$ matrix (with real coefficients) is invertible if and only if it is not the $0 so here, singular is not being taken in the sense of single, but rather in the sense of special, not common.

dvanderle

Ma dai! 26+ Fatti su Non Singular Matrix Definition With Example? The following matlab statements demonstrate the use of inv and verify that (ab)− 1 = b− 1a− 1 if a is a square nonsingular matrix of order n then its determinant is given by det(a) = a, a ∈ r.

Non Singular Matrix Definition With Example: An n × n matrix a is called nonsingular or invertible if there exists an n × n matrix b such that.

0 Response to "Ma dai! 26+ Fatti su Non Singular Matrix Definition With Example? The following matlab statements demonstrate the use of inv and verify that (ab)− 1 = b− 1a− 1 if a is a square nonsingular matrix of order n then its determinant is given by det(a) = a, a ∈ r."

Posting Komentar